On the afternoon of March 22, 2024, Wolfgang Steiner, a researcher at the French National Center for Scientific Research, was invited to give an online academic report entitled "Unique double base expansions and entropy of open dynamical systems".

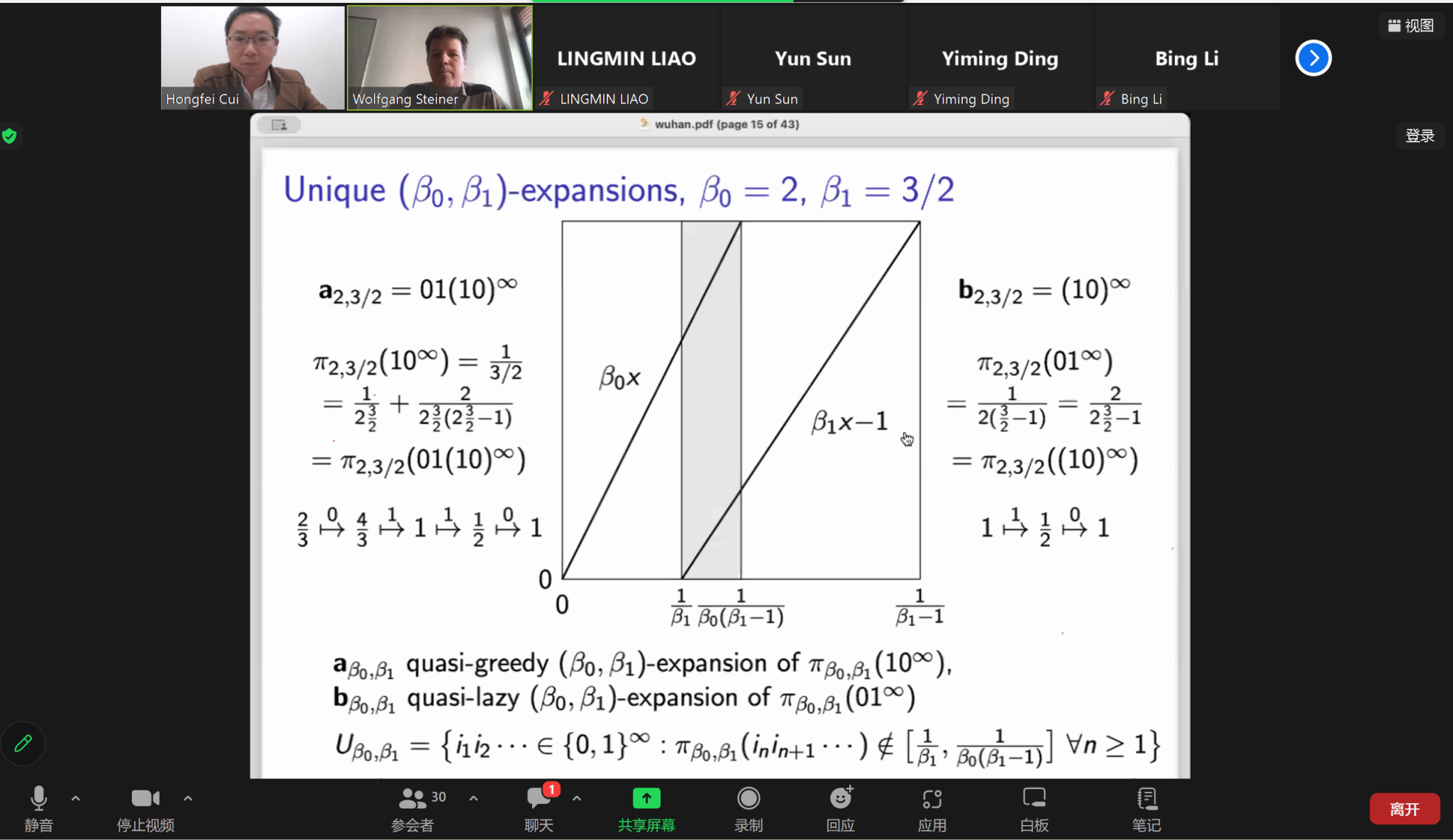
In the presentation, Prof. Steiner presented his latest research results in collaboration with mathematicians Vilmos Komornik and Yuru Zou. Starting from the (q0,q1) expansion of real numbers, the set that can be expanded uniquely (q0,q1) is introduced, and the specific expressions of G function and K function are given. where the G function can divide nontrivial sets and the K function can divide uncountable sets, and this result is visualized using images. subsequently

This paper describes the properties of the G function and the K function, including that these two functions are symmetrical with respect to q0 and q1, and are continuously decreasing, almost everywhere differentiable, and that the set of both the G function and the K function is satisfied with a Hausedorff dimension of 0. Finally, a simple characterization of the features of the S-adic sequence is proposed. The report attracted teachers and students from Wuhan University, Huazhong University of Science and Technology, South China University of Technology, Shenzhen University, Wuhan University of Technology and other schools.

About the Speaker:

Professor Wolfgang Steiner is a researcher at the French National Center for Scientific Research, currently engaged in number theory, dynamical systems and ergodic theory at the Institute of Fundamental Computing of the University of Paris-Cité, and has many profound results in the beta and alpha continuous fractional expansions related to number theory. He has published in many internationally renowned mathematics journals such as Adv. Math, Bull. Lond. Math. Soc., J. Eur. Math. Soc.(JEMS), Proc. Amer. Math. Soc., Trans. Amer. Math. Soc.; Ergodic Theory Dynam. Systems and others have published more than 50 articles.